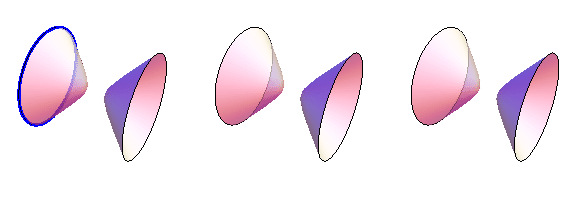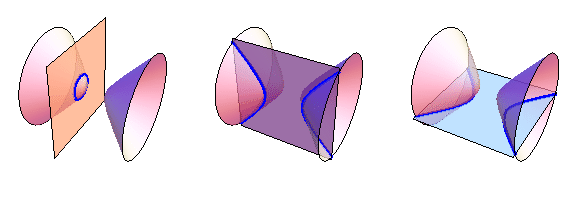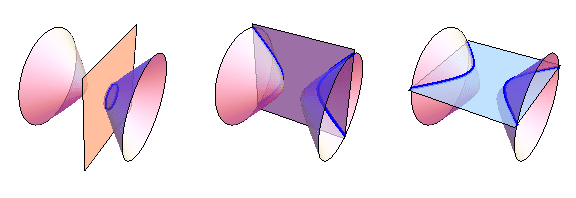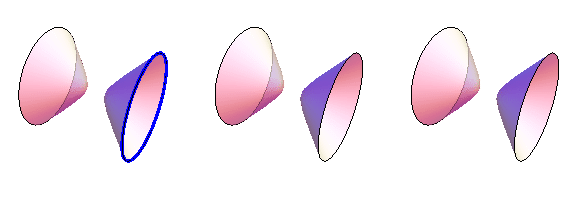The following are animations of the ,
, and
traces being taken of various surfaces.
- This is the sphere, defined by
. Notice that all three traces are families of circles. For the
traces, the radius of a circle in a given trace is
. This explains why there is no trace when
, then grow from
to
along
and then shrink from
back to
along
. By symmetry, identical properties hold when
is replaced by
or
.
- This is the hyperboloid of one sheet, defined by
. Notice that in the
and
traces, one sees a family of hyperbolas whereas in the
traces, one sees a family of circles.
- This is the hyperboloid of two sheets, defined by
. By contrast to the hyperboloid of one sheet, the
and
traces are families of hyperbolas, whereas the
traces are a family of circles. Notice that there is a region of
values for which the trace does not intersect the hyperboloids, corresponding the the non-existence of a solution to
when
is between
and
.